Related Pages
Pythagorean Triples
Types Of Triangles
Area Of Triangles
More Geometry Lessons
Recognizing Special Right Triangles
Recognizing special right triangles can provide ashortcut when answering some geometry questions. A special right triangle is a right trianglewhose sides are in a particular ratio, called the Pythagorean Triples. Youcan also use the Pythagorean theorem', but if you can see thatit is a special triangle it can save you some calculations.
A special right triangle is a right triangle with some regular feature that makes calculations on the triangle easier, or for which simple formulas exist. For example, a right triangle may have angles that form simple relationships, such as 45°–45°–90°. This is called an 'angle-based' right triangle. Special Right Triangles Chapter 8 Section 3 Learning Goal: Use properties of 45°-45 °-90 °, and 30 °-60 °-90 ° Triangles. Title: PowerPoint Presentation Author.
The following figures show some examples of special right triangles and Pythagorean Triples.Scroll down the page if you need more explanations about special right triangles, Pythagoreantriples, videos and worksheets.

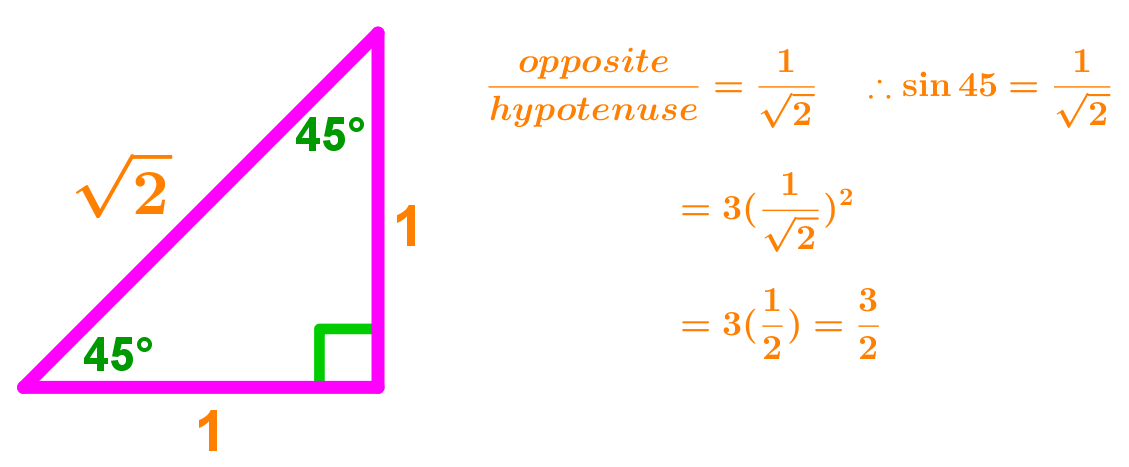
What is a 45°-45°-90° Triangle?
A 45°-45°-90° triangle is a special right triangle whose angles are 45°,45° and 90°. The lengths of the sides of a 45°-45°-90°triangle are in the ratio of 1 : 1 : √2.
A right triangle with two sides of equal lengths must be a 45°-45°-90° triangle.
You can also recognize a 45°-45°-90° triangle by the angles. A right trianglewith a 45° angle must be a 45°-45°-90° special right triangle.
Side1 : Side2 : Hypotenuse = x : x : x√2
Example 1:
Find the length of the hypotenuse of a right triangle if the lengths ofthe other two sides are both 3 inches.
Solution:
Step 1:This is a right triangle with two equal sides so it must be a 45°-45°-90°triangle.
Step 2:You are given that the both the sides are 3. If the first and second value of the ratiox:x:x√2 is 3 then the length of the third side is 3√2.
Answer: The length of the hypotenuse is 3√2 inches.
Example 2:
Find the lengths of the other two sides of a right triangle if the length of the hypotenuseis 4√2 inches and one of the angles is 45°.
Solution:
Step 1:This is a right triangle with a 45°-45°-90° triangle.
You are given that the hypotenuse is 4√2. Ifthe third value of the ratio n:n:n√2 is 4√2 then the lengths ofthe other two sides must 4.
Answer: Thelengths of the two sides are both 4 inches.
What is a 30°-60°-90° Triangle?
Another type of special right triangles is the 30°-60°-90°triangle. This is right triangle whose angles are 30°-60°-90°.The lengths of the sides of a 30°-60°-90°triangle are in the ratio of 1 : √3 : 2.
You can also recognize a 30°-60°-90° triangle by the angles. As long asyou know that one of the angles in the right-angle triangle is either 30°or 60° then it must be a 30°-60°-90° special right triangle. Aright triangle with a 30° angle or 60° angle must be a30°-60°-90° special right triangle.
Side1 : Side2 : Hypotenuse = x : x√3 : 2x
Example 1:
Find the length of the hypotenuse of a right triangle if the lengths ofthe other two sides are 4 inches and 4&dadic;3 inches.
Solution:
Step 1:Test the ratio of the lengths to see if it fits the n:n√2:2n ratio.
4:4√3:? = x:x√3:2x
Step 2:Yes, it is a 30°-60°-90° triangle for x = 4
Step 3:Calculate the third side.
2x = 2 × 4 = 8
Answer: Thelength of the hypotenuse is 8 inches.
Example 2:
Find the lengths of the other two sides of a right triangle if thelength of the hypotenuse is 8 inches and one of the angles is 30°.
Solution:
Step 1:This is a right triangle with a 30° angleso it must be a 30°-60°-90° triangle.
You are given that the hypotenuse is 8.
Substituting 8 into the third value of the ratio x:x√3:2x, we get that 2x = 8 ⇒ x = 4.
Substituting x = 4 into the first and second value of the ratio we get that the other twosides are 4 and 4√3.
Answer:The lengths of the two sides are 4 inches and 4√3 inches.
Special Triangles - Important Angles - 30°, 45°, 60°
45°-45°-90° Triangles, 30°-60°-90° Triangles.
- Show Video Lesson
Basic triangles you should know
The triangles are classified by side and by angle.
In this video you will learn:
- 3-4-5 triangles and similar triangles.
- 5-12-13 triangles and similar triangles.
- 45-45-90 right triangles.
- 30-60-90 triangles.
- equilateral triangles.
- relationship between equilateral and 30-60-90 triangles.
How to Solve Special Right Triangles?
When solving special right triangles,remember that a 30-60-90 triangle has a hypotenuse twice as longas one of the sides, and a 45-45-90 triangle has two equal sides.
- Show Video Lesson
Special Right Triangles in Geometry
45-45-90 and 30-60-90 degree triangles.
Discuss two special right triangles, how to derive the formulas tofind the lengths of the sides of the triangles by knowing the lengthof one side, and a few examples using them.

What are Pythagorean Triples?
Any group of 3 integer values that satisfies the equation:a2 + b2 = c2 is called a Pythagorean Triple.Any triangle that has sides that form a Pythagorean Triple must be a right triangle.Some examples of Pythagorean Triple triangles are: 3-4-5 Triangles and 5-12-13 Triangles.
What is a 3-4-5 Triangle?
A 3-4-5 triangle is right triangle whose lengths are in the ratioof 3:4:5. When you are given the lengths of two sides of a righttriangle, check the ratio of the lengths to see if it fits the3:4:5 ratio.
Side1 : Side2 : Hypotenuse = 3n : 4n : 5n
Example 1:
Find the length of the hypotenuse of aright triangle if the lengths of the other two sides are 6 inches and 8 inches.
Special Right Triangles Answer Key
Solution:
Step 1: Test the ratio of thelengths to see if it fits the 3n : 4n : 5n ratio.
6 : 8 : ? = 3(2) : 4(2) : ?
Step 2: Yes, it is a 3-4-5 triangle for n = 2.
Step 3: Calculate the third side.
5n = 5 × 2 = 10
Answer: The length of the hypotenuse is 10 inches.
Example 2:
Find the length of one side of a right triangle if the length of thehypotenuse is 15 inches and the length of the other side is 12 inches.
Solution:
Step 1: Test the ratio of the lengths to see if itfits the 3n : 4n : 5n ratio.
? : 12 : 15 = ? : 4(3) : 5(3)
Step 2: Yes, it is a 3-4-5 triangle for n = 3.
Step 3: Calculate the third side.
3n = 3 × 3 = 9
Answer: The length of the side is 9 inches.
What is a 5-12-13 Triangle?
A 5-12-13 triangle is a right-angled triangle whose lengths arein the ratio of 5:12:13. It is another example of a special righttriangle.
Example:
3-4-5 and 5-12-13 are examples of the PythagoreanTriple. They are usually written as (3, 4, 5) and (5, 12,13). In general, a Pythagorean triple consists of threepositive integers such that a2 + b2 = c2. Twoother commonly used Pythagorean Triples are (8, 15, 17) and (7,24, 25)
Concepts and patterns of Pythagorean triples
Examples and families of Pythagorean Triples
- Show Video Lesson
Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
Special Right Triangle: 30º-60º-90º MathBitsNotebook.com Topical Outline | Geometry Outline | MathBits' Teacher Resources Terms of Use Contact Person:Donna Roberts |
There are two 'special' right triangles that will continually appear throughout your study of mathematics: the 30º-60º-90º triangle and the 45º-45º-90º triangle. The special nature of these triangles is their ability to yield exact answers instead of decimal approximations when dealing with trigonometric functions. This page will deal with the 30º-60º-90º triangle.
All 30º-60º-90º triangles are similar! They satisfy Angle -Angle (AA) for proving trianlges similar. |
Congruent 30º-60º-90º triangles are formed when an altitude is drawn in an equilateral triangle. Remember that the altitude in an equilateral triangle will bisect the angle and is the perpendicular bisector of the side. If the side of the equilateral triangle is set to a length of 2 units, the Pythagorean Theorem will find the length of the altitude to be units. | |
Note: the side of the equilateral triangle need not be a length of 2 for the patterns to emerge. The choice of a side length of 2 simply makes the calculations easier. Yes, is smaller than 2, making the hypotenuse of 2 the longest side. Remember 2 can be written as .
| |
Once the sides of the 30º-60º-90º triangle are established, a series of relationships (patterns) can be identified between the sides of the triangle. ALL 30º-60º-90º triangles will possess these same patterns. These relationships will be referred to as 'short cut formulas' that can quickly answer questions regarding side lengths of 30º-60º-90º triangles, without having to apply any other strategies such as the Pythagorean Theorem or trigonometric functions. |
Since 30º-60º-90º triangles are similar, their corresponding sides are proportional. As such, we can establish a pattern as to how their sides are related. The following pattern formulas will let you quickly find the sides of a 30º-60º-90º triangle even when you are given only ONE side of the triangle. Remember, these formulas work ONLY in a 30º-60º-90º triangle!
|
|
Examples: |
This example shows the easiest application of the patterns (where the hypotenuse is given).
Always look at what is 'given' and what you need to find.
Find x and y. | x is the short leg (across from the 30º angle)x = ½ • 16 x = 8Answer | y is the long leg (across from the 60º angle)y = ½ • 16 • y = 8 Answer |
This example requires more thinking and more algebraic work (where the short leg is given).
Always start with what is 'given' and work from that point.
Find x and y. | 7 is the short leg (x is the hypotenuse) 7 = ½ • x x = 14 Answer | y is the long leg (use hypotenuse you found)y = ½ • 14 • y = 7 Answer |
This example requires the most algebraic and radical work (where long leg is a given integer).
Since the 'given' is the long leg, start with the long leg.
Find x and y. To remove a radical value from a denominator, multiply (top and bottom) by that radical value. Radical Review | y is the short leg (use hypotenuse you found)
|
Special Right Triangles 90-45-45
Notice that when you are working with a 30º-60º-90º triangle you are working with. Look at all of the THREEs at work! 30, 60, 90, When you work with 30º-60º-90º and 45º-45º-90º triangles, you will need to keep straight which radical goes with which triangle. |
I forgot the formula patterns! Now what? Remember that there is always more than one way to attack a problem! If you forget these formula patterns, you can use trigonometry functions and/or the Pythagorean Theorem to find the missing sides. Be warned, however, that the Pythagorean Theorem by itself, will not help you find both missing sides, since the Pythagorean Theorem needs two sides to work. |
NOTE: There-posting of materials(in part or whole) from this site to the Internet is copyright violation and is not considered 'fair use' for educators. Please read the 'Terms of Use'. |
Topical Outline | Geometry Outline | MathBitsNotebook.com | MathBits' Teacher Resources
Terms of Use Contact Person: Donna Roberts